Authors:
(1) Edward Crane, School of Mathematics, University of Bristol, BS8 1TH, UK;
(2) Stanislav Volkov, Centre for Mathematical Sciences, Lund University, Box 118 SE-22100, Lund, Sweden.
Table of Links
Reduction to the case of uniform geometry
All original points are eventually removed, a. s.
Acknowledgements and References
Abstract
We study a stochastic model of consensus formation, introduced in 2015 by Grinfeld, Volkov and Wade, who called it a multidimensional randomized Keynesian beauty contest. The model was generalized by Kennerberg and Volkov, who called their generalization the Jante’s law process. We consider a version of the model where the space of possible opinions is a convex body B in R d . N individuals in a population each hold a (multidimensional) opinion in B. Repeatedly, the individual whose opinion is furthest from the centre of mass of the N current opinions chooses a new opinion, sampled uniformly at random from B. Kennerberg and Volkov showed that the set of opinions that are not furthest from the centre of mass converges to a random limit point. We show that the distribution of the limit opinion is absolutely continuous, thus proving the conjecture made after Proposition 3.2 in Grinfeld et al.
Keywords: Jante’s law process, consensus formation, Keynesian beauty contest, rank-driven process, interacting particle system
Subject classification: 60J05, 60D05, secondary 60K35
1 Introduction
1.1 The multidimensional randomized Keynesian beauty contest

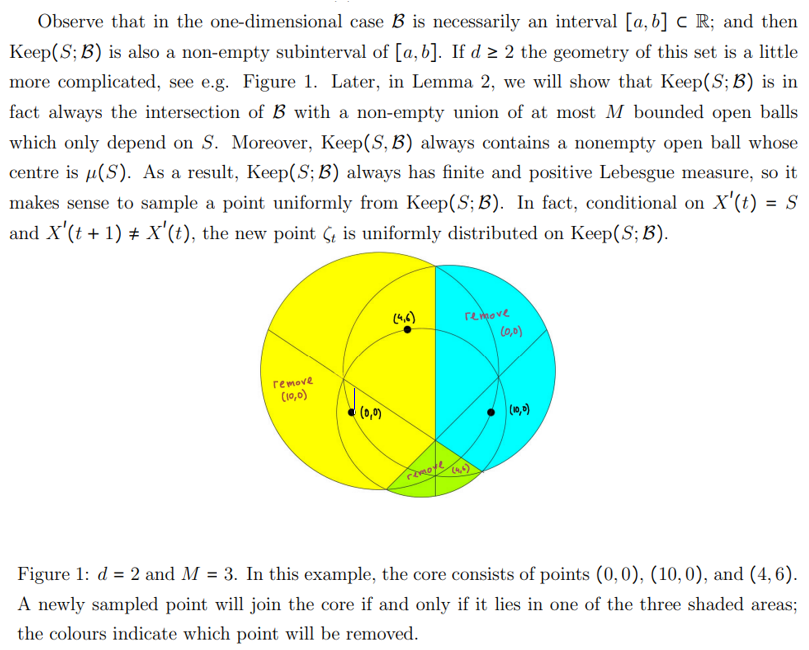


This paper is available on arxiv under CC 4.0 license.
1 The law of Jante is a literary caricature of the virtue in Scandinavian culture of not standing out from the crowd. It appeared in Aksel Sandemose’s satirical novel En flyktning krysser sitt spor, (A fugitive crosses his tracks), published in 1933.
2 Any other way of breaking ties would also be acceptable since a.s. ties do not occur.
3 The conjecture is stated immediately after [2, Proposition 3.2].
4 An analogous modification of the original process X(t) was already used as a tool in [2, §3.4].

